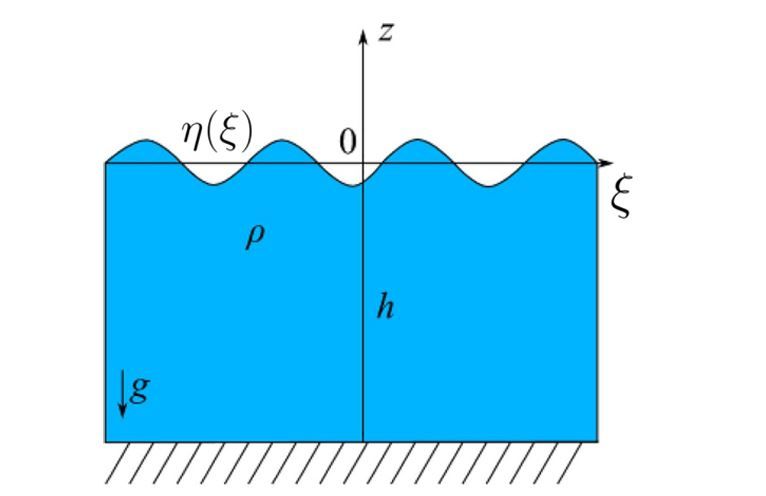
The Korteweg-de Vries (KdV) equation equation was one of the first integrable systems which caught the attention of many researchers during the 19th century due to its several distinguishing characteristics. On the one hand, it admitted a special kind of solution called a soliton: a solitary wave capable of traveling without losing its form and that whose interactions are elastical; on the other hand, the KdV equation exhibited a large quantity of independent conserved quantities regardless of its high nonlinearity. With this began the development of methods to characterise and study the KdV equation, a field which is still active nowadays and has evolved into the study of different integrable systems that share properties with the KdV equation, such as the sine-Gordon equation, the nonlinear Schrödinger equation or the Ablowitz-Kaup-Newell-Segur (AKNS) system.

One of the major breakthroughs in the study of the KdV equation came from Drinfeld and Sokolov in 1985, who showed there existed an algebraic structure underlying the KdV system associated to the recently famous Kac-Moody algebras. Moreover, beginning from an arbitrary affine Kac-Moody algebra, Drinfeld and Sokolov gave a method of constructing a hierarchy of evolution equations that could be solved under the same scheme under which the KdV equation could.

In this direction, the purpose of this work is to provide a fundamental background in which Drinfeld and Sokolov’s construction can be understood. To do this, we begin by introducing the KdV equation in a historical-oriented way, providing the main results of the theory which inspired the development of methods for solving the equation. Alongside, we introduce the theory of the Toda lattice and its generalisation, yet another similar system that is connected to the hierarchies proposed by Drinfeld and Sokolov.

After this, we exhibit a way of solving the KdV equation through the use of scattering methods of the Schrödinger equation. We then generalise this method by the means of operators in a Hilbert space, method which nowadays is known as the Lax scheme. In this scheme, a nonlinear evolution equation is expressed as a compatibility condition for the time evolution of the eigenfunctions of a linear operator. We then show how this method gives rise to an infinite sequence of conserved quantities for the KdV equation.

To finish, we introduce the Zakharov-Shabat scheme, a framework that generalises the Lax scheme and provides a way of interpreting nonlinear evolution equations as a pair of evolution equations given by a pair of matrix-valued function. By generalising these matrices to an abstract Kac-Moody algebra we are then able to construct the hierarchies proposed by Drinfeld and Sokolov. We conclude by exhibiting how one of the hierarchies of Drinfeld and Sokolov can be used to described flows of the generalised Toda lattice.